Making Sense
Submitted by
Margaret Williams
Teaching and Learning Specialist for Intermediate Mathematics
Anoka-Hennepin ISD 11
Greetings from Anoka! This weather has been amazing, right? It’s been like a winter vacation without having to leave town. Well, and without leaving work.
We have continued to look at rich tasks and how to “do it better”.
Lessons learned:
Addressing Misconceptions – Strategy sharing is a great time to address misconceptions. In a recent second grade math class, we were looking at student work around a Compare Difference Unknown task:
Addi had 84 gumballs. Kate had 29 gumballs. How many more gumballs did Addi have than Kate?:
Two misconceptions appeared: Some students had a difficult time understanding the task (compare problems tend to be challenging for younger students as there is no action. There is nothing in the problem itself that tells them how to approach the task. Many students end up adding the two quantities together rather than finding the difference between the two. The other misconception was the notion that “I can’t subtract 9 from 4, so I’ll subtract 4 from 9.” In planning a re-engagement lesson with this problem, we decided we wanted to address the latter idea. However, when we began the whole group discussion, it became clear we had to come to consensus about the meaning of the problem. Lesson learned: Understanding the problem is critical. If they don’t understand the task, it’s going to be difficult to discuss strategies. Possible solution to more challenging problem types would be to create a context that has an implied action the children can visualize. For Compare Difference Unknown problems, consider contexts that have subjects that are commonly matched – such as dogs and collars, chairs and desks, kids and hats, etc.
Anonymous Sharing – Keeping students’ names hidden during sharing worked better than we expected. We noticed there was greater participation because it promoted the next Lesson.
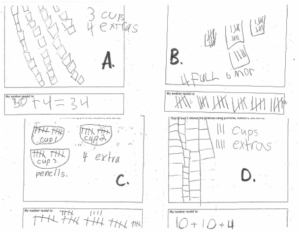
Students’ Interpreting Each Other’s Work – This was AWESOME! Below is an example of four children’s thinking from a first grade classroom in one of our school-wide Title One schools. The problem the children had to solve was:
Your teacher had 34 pencils. She put them into cups that held 10 pencils each. How many full cups did she have? Were there extras? How many?
Strategies A, C and D were pretty similar, whether the first graders used tallies or snap cubes. The young mathematicians built tens out of ones, as directed by the problem. The equations need a little work, but just looking at what the students are doing gives you information about what they understand at that point in time. There is certainly some promise there. Hmmm…Look at Strategy B. What’s going on there? The child wrote “4 full” and “6 more” We can go back to that age old question – Is he wrong or did he answer a different question?
As adults, we can figure out that the student is likely indicating that with 6 more pencils, the teacher will have 4 full cups. The other students struggled with making sense of B’s response. They wanted to understand and they tried to explain the strategy…unsuccessfully. This was a wonderful opportunity for discussions around making sense of the problem, communicating clearly and making sense of each other’s thinking. Yes, in first grade. We work on this in kindergarten, as well. Making sense is likely the most important thing one does in the math classroom and it should be an expectation from Day 1.
I’m interested in hearing your experiences with classroom discussions. What are your challenges? What structures and strategies have produced successful conversations? Please send me your thoughts!
Hope to see you in Duluth for the MCTM Spring Conference!
Margaret Williams
Want to connect?
margaret.williams@ahschools.us or @nanstermath
New and Future Teachers: Be sure to attend the CONNECT Session on the Thursday evening before the MCTM Spring Conference in Duluth. For more information, contact connect@mctm.org<mailto:connect@mctm.org>.